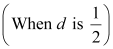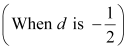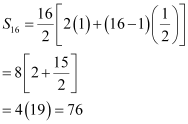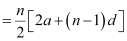Question 1:
Which term of the A.P. 121, 117, 113 … is its first negative term?
[Hint: Find n for an < 0]
Given A.P. is 121, 117, 113 …
a = 121
d = 117 − 121 = −4
an = a + (n − 1) d
= 121 + (n − 1) (−4)
= 121 − 4n + 4
= 125 − 4n
We have to find the first negative term of this A.P.
Therefore, 32nd term will be the first negative term of this A.P.
a = 121
d = 117 − 121 = −4
an = a + (n − 1) d
= 121 + (n − 1) (−4)
= 121 − 4n + 4
= 125 − 4n
We have to find the first negative term of this A.P.
Therefore, 32nd term will be the first negative term of this A.P.
Question 2:
The sum of the third and the seventh terms of an A.P is 6 and their product is 8. Find the sum of first sixteen terms of the A.P.
We know that,
an = a + (n − 1) d
a3 = a + (3 − 1) d
a3 = a + 2d
Similarly, a7 = a + 6d
Given that, a3 + a7 = 6
(a + 2d) + (a + 6d) = 6
2a + 8d = 6
a + 4d = 3
a = 3 − 4d (i)
Also, it is given that (a3) × (a7) = 8
(a + 2d) × (a + 6d) = 8
From equation (i),
From equation (i),
an = a + (n − 1) d
a3 = a + (3 − 1) d
a3 = a + 2d
Similarly, a7 = a + 6d
Given that, a3 + a7 = 6
(a + 2d) + (a + 6d) = 6
2a + 8d = 6
a + 4d = 3
a = 3 − 4d (i)
Also, it is given that (a3) × (a7) = 8
(a + 2d) × (a + 6d) = 8
From equation (i),
From equation (i),
Question 3:
A ladder has rungs 25 cm apart. (See figure). The rungs decrease uniformly in length from 45 cm at the bottom to 25 cm at the top. If the top and bottom rungs are
m apart, what is the length of the wood required for the rungs?
[Hint: number of rungs
]
It is given that the rungs are 25 cm apart and the top and bottom rungs are
m apart.
∴ Total number of rungs
Now, as the lengths of the rungs decrease uniformly, they will be in an A.P.
The length of the wood required for the rungs equals the sum of all the terms of this A.P.
First term, a = 45
Last term, l = 25
n = 11
Therefore, the length of the wood required for the rungs is 385 cm.
∴ Total number of rungs
Now, as the lengths of the rungs decrease uniformly, they will be in an A.P.
The length of the wood required for the rungs equals the sum of all the terms of this A.P.
First term, a = 45
Last term, l = 25
n = 11
Therefore, the length of the wood required for the rungs is 385 cm.
Question 4:
The houses of a row are number consecutively from 1 to 49. Show that there is a value of x such that the sum of numbers of the houses preceding the house numbered x is equal to the sum of the number of houses following it.
Find this value of x.
[Hint Sx − 1 = S49 − Sx]
The number of houses was
1, 2, 3 … 49
It can be observed that the number of houses are in an A.P. having a as 1 and d also as 1.
Let us assume that the number of xth house was like this.
We know that,
Sum of n terms in an A.P.
Sum of number of houses preceding xth house = Sx − 1
Sum of number of houses following xth house = S49 − Sx
It is given that these sums are equal to each other.
However, the house numbers are positive integers.
The value of x will be 35 only.
Therefore, house number 35 is such that the sum of the numbers of houses preceding the house numbered 35 is equal to the sum of the numbers of the houses following it.
1, 2, 3 … 49
It can be observed that the number of houses are in an A.P. having a as 1 and d also as 1.
Let us assume that the number of xth house was like this.
We know that,
Sum of n terms in an A.P.
Sum of number of houses preceding xth house = Sx − 1
Sum of number of houses following xth house = S49 − Sx
It is given that these sums are equal to each other.
However, the house numbers are positive integers.
The value of x will be 35 only.
Therefore, house number 35 is such that the sum of the numbers of houses preceding the house numbered 35 is equal to the sum of the numbers of the houses following it.
Question 5:
A small terrace at a football ground comprises of 15 steps each of which is 50 m long and built of solid concrete.
Each step has a rise of 1/4 m and a tread of 1/2 m (See figure) calculate the total volume of concrete required to build the terrace.
From the figure, it can be observed that
1st step is 1/2 m wide,
2nd step is 1 m wide,
3rd step is 3/2 m wide.
Therefore, the width of each step is increasing by 1/2 m each time whereas their height 1/4 m and length 50 m remains the same.
Therefore, the widths of these steps are
Volume of concrete in 1st step
Volume of concrete in 2nd step
Volume of concrete in 3rd step
Volume of concrete required to build the terrace is 750 m3.